 login/create account
login/create account
Monochromatic empty triangles
If 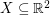 is a finite set of points which is 2-colored, an empty triangle is a set
is a finite set of points which is 2-colored, an empty triangle is a set 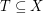 with
with 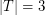 so that the convex hull of
so that the convex hull of 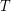 is disjoint from
is disjoint from  . We say that
. We say that 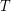 is monochromatic if all points in
is monochromatic if all points in 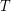 are the same color.
are the same color.
 with the following property. If
with the following property. If 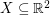 is a set of
is a set of  points in general position which is 2-colored, then it has
points in general position which is 2-colored, then it has 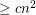 monochromatic empty triangles.
monochromatic empty triangles. It is known that any set of  points in the plane in general position contains
points in the plane in general position contains 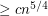 monochromatic empty triangles.
monochromatic empty triangles.
Bibliography
* indicates original appearance(s) of problem.
Yes indeed. However in this
Yes indeed. However in this types of problems it is generally implied that the statement is for a sufficently large n.
Original source and one improvement.
The conjecture appeared first in "Oswin Aichholzer, Ruy Fabila-Monroy, David Flores-Peñaloza, Thomas Hackl, Clemens Huemer, and Jorge Urrutia. Empty monochromatic triangles. In Proceedings of the 20th Canadian Conference on Computational Geometry (CCCG2008), pages 75-78, 2008."
In this paper the authors show that any set of n points in general position has 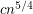 empty monochromatic triangles. You can get this paper from http://cccg.ca/proceedings/2008/paper18.pdf
empty monochromatic triangles. You can get this paper from http://cccg.ca/proceedings/2008/paper18.pdf
There is one improvement showing that any set of n points in general position has 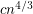 empty monochromatic triangles in: "J. Pach, G. Toth. Monochromatic empty triangles in two-colored point sets. In: Geometry, Games, Graphs and Education: the Joe Malkevitch Festschrift (S. Garfunkel, R. Nath, eds.), COMAP, Bedford, MA, 2008, 195--198." Get it from: http://www.math.nyu.edu/~pach/publications/emptytriangle102408.pdf
empty monochromatic triangles in: "J. Pach, G. Toth. Monochromatic empty triangles in two-colored point sets. In: Geometry, Games, Graphs and Education: the Joe Malkevitch Festschrift (S. Garfunkel, R. Nath, eds.), COMAP, Bedford, MA, 2008, 195--198." Get it from: http://www.math.nyu.edu/~pach/publications/emptytriangle102408.pdf
The lower bound has been improved
The lower bound has been improved to cn4/3.
J. Pach and G. Toth: Monochromatic empty triangles in two-colored point sets, in: Geometry, Games, Graphs and Education: the Joe Malkevitch Festschrift (S. Garfunkel, R. Nath, eds.), COMAP, Bedford, MA, 2008, 195--198.

 Drupal
Drupal CSI of Charles University
CSI of Charles University
This has a trivial
This has a trivial counterexample for c > 0.
Consider X = {(0,0), (0,1), (1,0)}, colored {red, blue, blue} respectively. There is only one empty triangle in X, and it is not monochromatic. So it has 0 monochromatic empty triangles, and 0 is not > c*(3^2) for c > 0.