Home » Keyword index

coloring


Graph Theory » Topological G.T. » Crossing numbers

Crossing numbers and coloring ★★★

Author(s): Albertson

We let 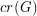$ cr(G) $ denote the crossing number of a graph $ G $.

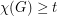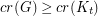Conjecture   Every graph $ G $ with $ \chi(G) \ge t $ satisfies $ cr(G) \ge cr(K_t) $.

Keywords: coloring; complete graph; crossing number

Posted by mdevos
updated September 4th, 2009
add new comment
Graph Theory » Coloring » Vertex coloring

Are vertex minor closed classes chi-bounded? ★★

Author(s): Geelen

Question   Is every proper vertex-minor closed class of graphs chi-bounded?

Keywords: chi-bounded; circle graph; coloring; vertex minor

Posted by mdevos
updated May 16th, 2009
add new comment
Graph Theory » Coloring » Vertex coloring

Graphs with a forbidden induced tree are chi-bounded ★★★

Author(s): Gyarfas

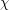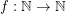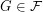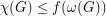Say that a family $ {\mathcal F} $ of graphs is $ \chi $-bounded if there exists a function $ f: {\mathbb N} \rightarrow {\mathbb N} $ so that every $ G \in {\mathcal F} $ satisfies $ \chi(G) \le f (\omega(G)) $.

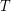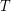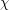Conjecture   For every fixed tree $ T $, the family of graphs with no induced subgraph isomorphic to $ T $ is $ \chi $-bounded.

Keywords: chi-bounded; coloring; excluded subgraph; tree

Posted by mdevos
updated May 16th, 2009
add new comment
Graph Theory » Topological G.T.

Domination in plane triangulations ★★

Author(s): Matheson; Tarjan

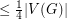Conjecture   Every sufficiently large plane triangulation $ G $ has a dominating set of size $ \le \frac{1}{4} |V(G)| $.

Keywords: coloring; domination; multigrid; planar graph; triangulation

Posted by mdevos
updated May 4th, 2009
add new comment
Graph Theory » Coloring » Vertex coloring

Double-critical graph conjecture ★★

Author(s): Erdos; Lovasz

A connected simple graph $ G $ is called double-critical, if removing any pair of adjacent vertexes lowers the chromatic number by two.

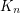Conjecture   $ K_n $ is the only $ n $-chromatic double-critical graph

Keywords: coloring; complete graph

Posted by DFR
updated November 30th, 2009
2 comments
Graph Theory » Coloring » Vertex coloring

Counting 3-colorings of the hex lattice ★★

Author(s): Thomassen

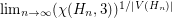Problem   Find $ \lim_{n \rightarrow \infty} (\chi( H_n , 3)) ^{ 1 / |V(H_n)| } $.

Keywords: coloring; Lieb's Ice Constant; tiling; torus

Posted by mdevos
updated July 5th, 2008
add new comment
Graph Theory » Coloring

4-regular 4-chromatic graphs of high girth ★★

Author(s): Grunbaum

Problem   Do there exist 4-regular 4-chromatic graphs of arbitrarily high girth?

Keywords: coloring; girth

Posted by mdevos
updated January 29th, 2012
2 comments
Graph Theory » Probabilistic G.T.

Coloring random subgraphs ★★

Author(s): Bukh

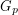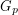If $ G $ is a graph and $ p \in [0,1] $, we let $ G_p $ denote a subgraph of $ G $ where each edge of $ G $ appears in $ G_p $ with independently with probability $ p $.

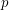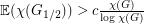Problem   Does there exist a constant $ c $ so that $ {\mathbb E}(\chi(G_{1/2})) > c \frac{\chi(G)}{\log \chi(G)} $?

Keywords: coloring; random graph

Posted by mdevos
updated June 4th, 2009
1 comment
Graph Theory » Coloring » Vertex coloring

Hedetniemi's Conjecture ★★★

Author(s): Hedetniemi

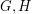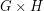Conjecture   If $ G,H $ are simple finite graphs, then $ \chi(G \times H) = \min \{ \chi(G), \chi(H) \} $.

Here $ G \times H $ is the tensor product (also called the direct or categorical product) of $ G $ and $ H $.

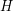Keywords: categorical product; coloring; homomorphism; tensor product

Posted by mdevos
updated March 1st, 2020
5 comments
Graph Theory » Topological G.T. » Coloring

Degenerate colorings of planar graphs ★★★

Author(s): Borodin

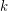A graph $ G $ is $ k $-degenerate if every subgraph of $ G $ has a vertex of degree $ \le k $.

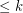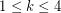Conjecture   Every simple planar graph has a 5-coloring so that for $ 1 \le k \le 4 $, the union of any 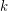$ k $ color classes induces a 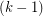$ (k-1) $-degenerate graph.

Keywords: coloring; degenerate; planar

Posted by mdevos
updated May 21st, 2008
add new comment
Syndicate content